Ìàòåìàòèêà / 5. Ìàòåìàòè÷åñêîå ìîäåëèðîâàíèå
Romanuke V. V., c. t. s., associate professor
Khmelnytskyy
National University, Ukraine
Five variants of
generating nonregular projector optimal strategy in a three-dimensional problem
of minimizing maximal imbalance for removing uncertainties and their
relationships to start finding projector optimal behaviors continuum
Surely that every
mathematical model is encircled with uncertainties of its parameters. Speaking
more globally, there always are several similar but different mathematical
models to an event or process and the problem of selecting the single model
begets uncertainty. Actually, there may be underlined the following categories
of the uncertainty origins: the uncertainty of the parameter, defined on a set
of values, the uncertainty of the function, defined on a functional set, and
the uncertainty of the mathematical model, defined on a set of mathematical
models of the definite class, describing the same event or process. It is clear
that the uncertainty of the parameter, given either in scalar or vector form,
is the lightest problem to be solved, using a decision making theory model or
game modeling. However, even the simplest antagonistic game models are tinted
with a lot of peculiarities, becoming apparent only within special conditions.
As a pattern to the said, there is a three-dimensional problem of minimizing
maximal imbalance for removing uncertainties [1] as the defined on the
hyperparallelepiped
(1)
antagonistic game,
having the kernel
(2)
as the function of
the pure strategies 
of the first player
and of the pure strategies 
of the second player,
where 
. Here the optimal game value is found as
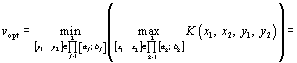
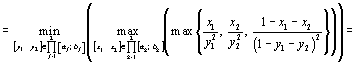
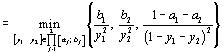. (3)
For most cases the optimal
behavior of the second player (projector) 
is determined by the
roots 
of the relationship
[1, p. 19]
, (4)
giving the optimal
game value (3) and projector optimal behavior regular components
, 
. (5)
But that regularity
may be disregarded if one of the following is true:
by 
, (6)
, (7)
by 
, (8)
, (9)
, 
by 
and 
for 
. (10)
Those variants (6) — (10) generate nonregular projector optimal strategy
as they stipulate the corresponding inequalities instead of (4):
, (11)
, 
, (12)
, (13)
, 
, (14)
and
, 
,
, 
(15)
by 
and 
for 
. Clearly that equalizing the inequalities (11) — (15) in finding
projector optimal behavior will give continuum of its optimal strategies in
every of the disclosed five variants.
References
1. Ðîìàíþê Â. Â. Ìîäåëþâàííÿ 䳿 íîðìîâàíîãî îäèíè÷íîãî íàâàíòàæåííÿ íà òðè êîëîíè îäíàêîâî¿ âèñîòè ó áóä³âåëüí³é êîíñòðóêö³¿ ³ çíàõîäæåííÿ îïòèìàëüíî¿ ïëîù³ êîæíî¿ îïîðè / Â. Â. Ðîìàíþê // Ïðîáëåìè òðèáîëî㳿. — 2010. — ¹ 3. — Ñ. 18 — 25.