ÌÀÒÅÌÀÒÈÊÀ/1.Äèôôåðåíöèàëüíûå è èíòåãðàëüíûå óðàâíåíèÿ
Dokukova
N.A., Kaftaikina E.N. Konon P. N.
Belarusian State University, Belarus
ONE METHOD FOR SOLVING THE DYNAMICS OF BENDING AND TWISTING OF THE RING
Introduction. A comparative analysis of technique numerically-analytical calculation of
the stress-deformed state loaded into the plane of the ring and the twisted
cross-section, irregularly distributed points for given initial and boundary
conditions, which take into account the periodicity of solutions to the
circumferential coordinate, proposed by the authors, represented at the
article, using known solutions of the problem of bending-torsional vibrations
of the ring. Well known that classical techniques can only detect vibrations of its own and
the natural frequencies of the closed
area of a solid body.
The developed technique fully
integrates mixed problem, can
effectively find an exact
solution of a certain type of
mathematical physics.
The
problem of bending and
torsion of the ring plane of its cross-sectional is considered [1]. The
ring is inextensible circular beam of
constant cross section with a radius R. Position
of the ring cross-section during the movement is characterized by
shifting x3 its center of gravity from the ring plane and
the angle of rotation of the section x4 (fig. 1a).
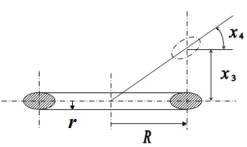
a b
Figure 1 - Scheme of the stress state of the ring (a -
section of the ring, b - beam segment of
the 
length)
Bending, twisting moments and shear force perpendicular to the plane of the ring are appeared in the cross section of a circular beam (fig. 1b). Bending and twisting
moments in each of the cross
sections are determined by summing
all the power factors.
, 
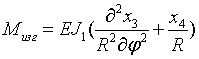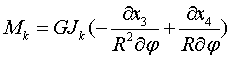(1)
The
system of equilibrium equations of the ring is made on the basis of the equations of moments about the normal n and tangent
to the axis of beam segment (fig. 1 b) [1]:
, 
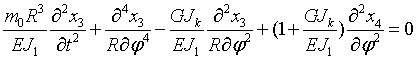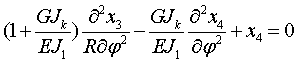(2)
Provide the known standard solution of differential equations in partial derivatives (2) in the form of
Fourier series [1, 2]
, 
. (3)
Substituting the values of x3(j,t)
and x4(j,t)
in the equations of motion (2), and equalization to zero of the resulting system determinant
of algebraic equations allows to
find the natural frequencies, the
smallest (the second one) of which is nonzero:
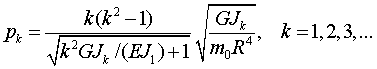. (4)
For the known and found physical
parameters: E = 2.1·1011 N/m2, G = 8.0·1010
N/m2, J1 = 2.0·10-5 m4, Jk
= 7.95·10-8 m4, m0 = 6.5 kg/m, R = 0.15 m, r
= 0.015 m, - the second and the
smallest frequency p2 has the following value: 8316,28 rad/sec. Solution (3)
is represented as the following [1]:
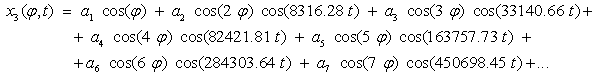(5)
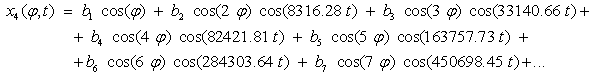(6)
The rate of displacement of the circular beam center line and the
angular rotation of the section, perpendicular
to it, changes, are proportional to the
natural frequencies, accelerations are proportional to
the squares of natural frequencies.
This adverse factor should be considered in a full statement of the problem to determine the corresponding coefficients of series
(5) and (6). In
order to have significant problem solution satisfying the physical sense, the coefficients should
be set 
for k
> 2. In this case an
approximate solution of the natural oscillations can
obtained (3):
(7)
Consider the problem solution (2) with
specific right part using methods, developed at the jobs [3, 4]. To do this, reduce
the system of equations (2) to
such a canonical form:
, 
(8)
Define the initial and boundary conditions, not
inconsistent with the physical nature of the problem
(9)
,
, (10)
, 
,
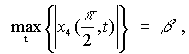
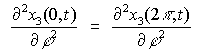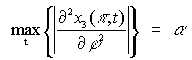where 
, 
, 
, 
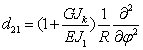- differential operators; k - natural numbers; a = 6.2, b = -6.2 - dimensionless amplitude of
the external load
torque distributed along a circular beam (fig. 2); q1 , q2 - arbitrary constants; a = 10-7 ì; b = 1.179 10-4 Rad;
p = 31.42 Rad/sec - frequency of
forced oscillations of external influences, unevenly distribution of along the midline of the ring.
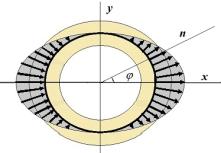
Figure
2 - Plot of the total load
torque distributed in the transverse sections of a circular rod (ring)
Apply differential operators L2 and L1 consequentially to
equations (8) and use properties of their linear transposition, obtain new system of differential equations in partial derivatives, in which each
of the equations independently of the other and has only one unknown function x3(j,t)
or x4(j,t)
, (11)
.
In the classical notation
system of equations (11) takes
the following form:
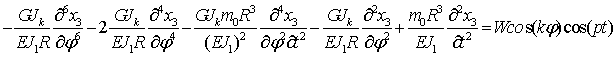, (12)
.
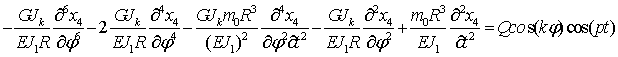Here new amplitudes have the following values: W
= 0.34·10-7, Q = 0.3221·10-4.
According to the general rules of the method
of separation of variables for partial differential equations, find solutions
of the homogeneous equations of mathematical
physics in the form:
,
, (13)
joined
by special solutions
of x
(j,t) or x
(j,t), containing a harmonic
function of the right sides of (13). General solutions will consist of superpositions of these solutions
,
. (14)
The
initial problem (9) to determine
the function 
, in
which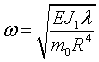 
- natural
frequency, the integrating factor l - unknown parameter to be determined from an
analysis of possible solutions to
the homogeneous differential equation for determining the middle line forms a circular ring and
the boundary problem (10). Express the general
equation of (12) in components 
, 
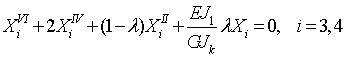, (15)
The
condition for choosing
the parameter l can be used algebraic stability
criteria of Voronov V.S. [5], providing a stable form of natural
vibrations
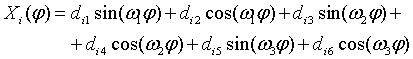. (16)
Under
the assumptions made about
the physical parameters of magnitude
l less
than 
. All frequencies of the
modes of vibration are in
the range 
, and the natural frequency are in the range - 
Rad/sec. The range for 
is well agreed
with the solution (7), presented in [1]. The
general solution of the problem (2), (9), (10) are functions of the form:
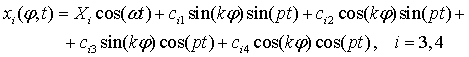. (17)
For
k = 1, l = 0.0002
, a
= 1.5, b = - 1.5 and initial
conditions a = 10-7 m, b = 1.179 10-4 Rad exact
solution is obtained (8), (9), (10)
in the form:
(18)
The
found solution fully satisfies
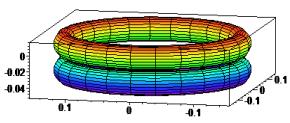
the boundary conditions (9), (10) of the set problem and presented in fig. 3-4.
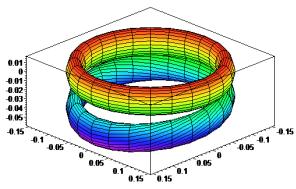
a b
Figure 3 - Forced displacement from the equilibrium
position of the ring and twisting
its cross-sections at a time t = 0.01 sec (a), t = 10.01 sec (b)
a b
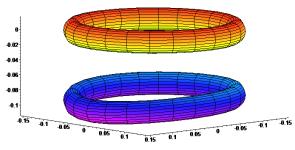
Figure 4 - Natural oscillations of the displacement from the
equilibrium position of the ring and
twisting its cross-sections
at a time t =0.01 sec
(a), t = 10.01 sec (b)
For
k = 3, l = 0.0002
, a
= 0.21, b = - 0.0093 , and initial
conditions a = 10-7 m, b = 1.179 10-4 Rad exact solution is obtained (8), (9), (10) â
âèäå:
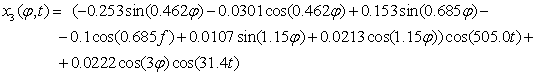(20)
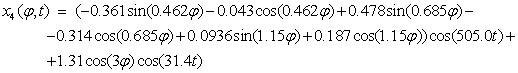
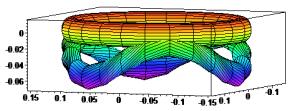
a b
Figure 5 - Forced displacement from the equilibrium
position of the ring and twisting
its cross-sections at a time t =
0.01sec (a), t = 10.01 sec (b)
Conclusion: The developed method of
studying the stress-deformed state in conjunction with the dynamic displacements of elastic elements with a consistent application of differential operators, allows to omit the tedious mathematical
calculations, to replace a system of two coupled partial differential equations
of second and fourth order in two independent non-homogeneous
differential equations of mathematical physics, 6-th order with
constant coefficients. The method can be extended to other mechanical objects
with complex environments and structures to the
challenges of higher orders of
complexity.
References
1.Biderman,V.L. The theory of mechanical
vibrations.-Ì.:Vyssh.shkola.1980. 408 p.
2.Moiseev,N.N. Asymptotic Methods in Nonlinear Mechanics-Ì.:Nauka.1969.380
p.
3. Vysotsky, M.S., Dokukova, N.A., Konon, P.N.
Some features of the mechanical oscillatory systems to
two degrees of freedom//Vestnik of
the Foundation for Fundamental Research. - 2006. - ¹ 1 p.78-85.
4. Dokukova, N.A. and
Konon, P.N. General laws governing in mechanical vibratory systems//Journal of
Engineering Physics and Thermophysics, 2006, Volume 79, Number 4, Pages
824-831, Publisher Springer New York, ISSN: 1062-0125.
5. Voronov, V. S. Sustainability indicators of robust control systems//Izv. RAN. Theory and Control Systems. 1995.
¹ 6. p.
49-54.